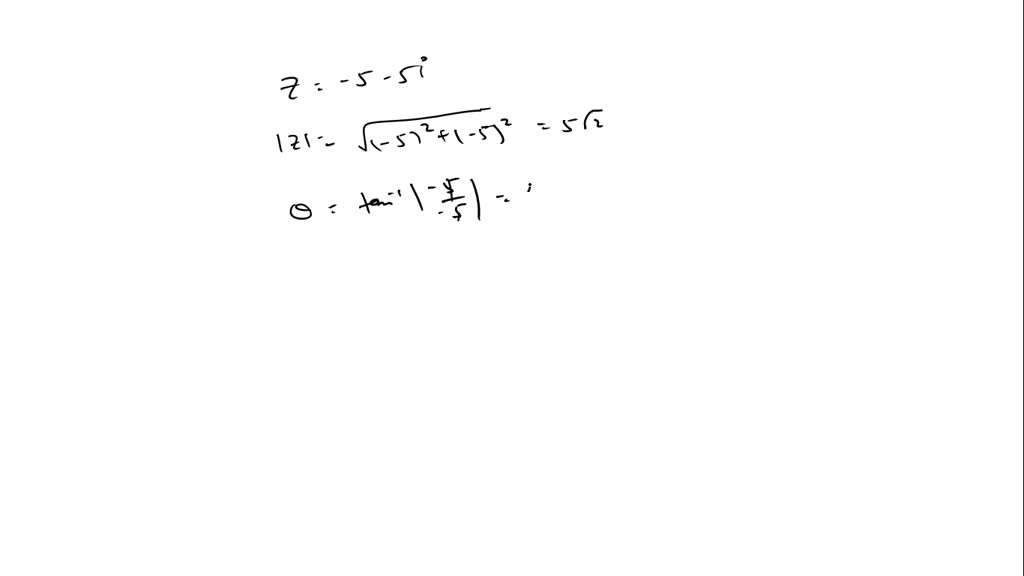Express The Complex Number In Trigonometric Form
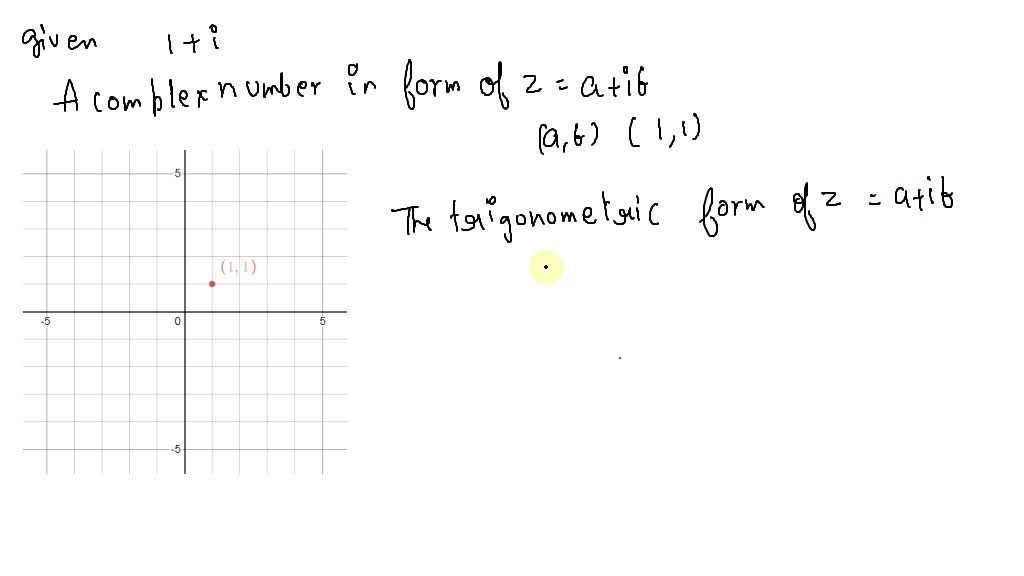
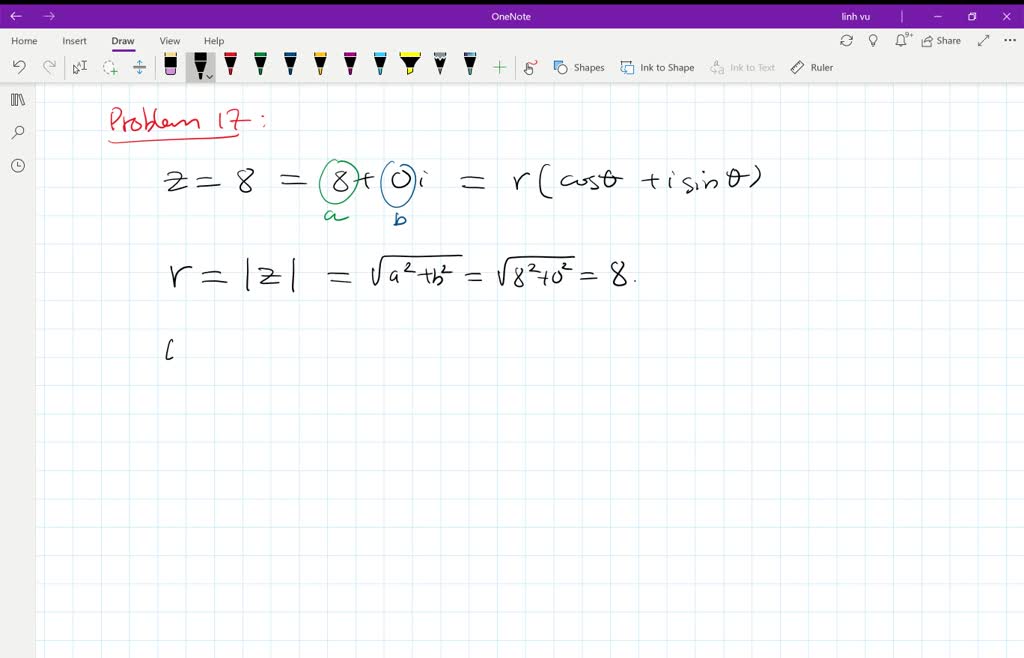
Express The Complex Number In Trigonometric Form - As a refresher, the distance between the origin and the complex number is equal to $|a + bi| = \sqrt{a^2 + b^2}$. Web the trigonometric form of a complex number z = a + bi is = r(cos i sin ); Get this answer verified by an expert. Web express the complex number in trigonometric form. To convert a complex number z to trigonometric form, we use the formula: Web represent the complex number 5 + 7 i graphically and express it in its polar form. Web the second chapter is devoted to the study of the trigonometric form of complex numbers and it contains two sections dealing with the following aspects: To do this, we first determine the. How do you express the complex number in trigonometric form: Web how do you express the complex number in trigonometric form:
Next, we must determine the argument θ. To convert a complex number z to trigonometric form, we use the formula: Web take the following complex number in rectangular form. Web represent the complex number 5 + 7 i graphically and express it in its polar form. As a refresher, the distance between the origin and the complex number is equal to $|a + bi| = \sqrt{a^2 + b^2}$. This is the trigonometric form of a complex number where |z| | z | is the modulus and θ θ is the angle created on the. R = 5 2 + 7 2 =. How do you express the complex number in trigonometric form: = b is called the argument of z. Distributing to terms inside the (.
As a refresher, the distance between the origin and the complex number is equal to $|a + bi| = \sqrt{a^2 + b^2}$. Web the second chapter is devoted to the study of the trigonometric form of complex numbers and it contains two sections dealing with the following aspects: Web express the complex number in trigonometric form. Web represent the complex number 5 + 7 i graphically and express it in its polar form. Web the trigonometric form of a complex number z = a + bi is = r(cos i sin ); All of this can also be thought of in terms. How do you express the complex number in trigonometric form: Web express the complex number in trigonometric form. Web the polar form of a complex number expresses a number in terms of an angle \(\theta\) and its distance from the origin \(r\). To do this, we first determine the.
Trigonometric Form of a Complex Number Represent
To convert a complex number z to trigonometric form, we use the formula: 6 (cos (2pi/3) + i sin (2pi/3)) arrow right. Choose the notation your class is using. Given a complex number, z = a + b i, we first compute the modulus, r = a 2 + b 2. Web the polar form of a complex number expresses.
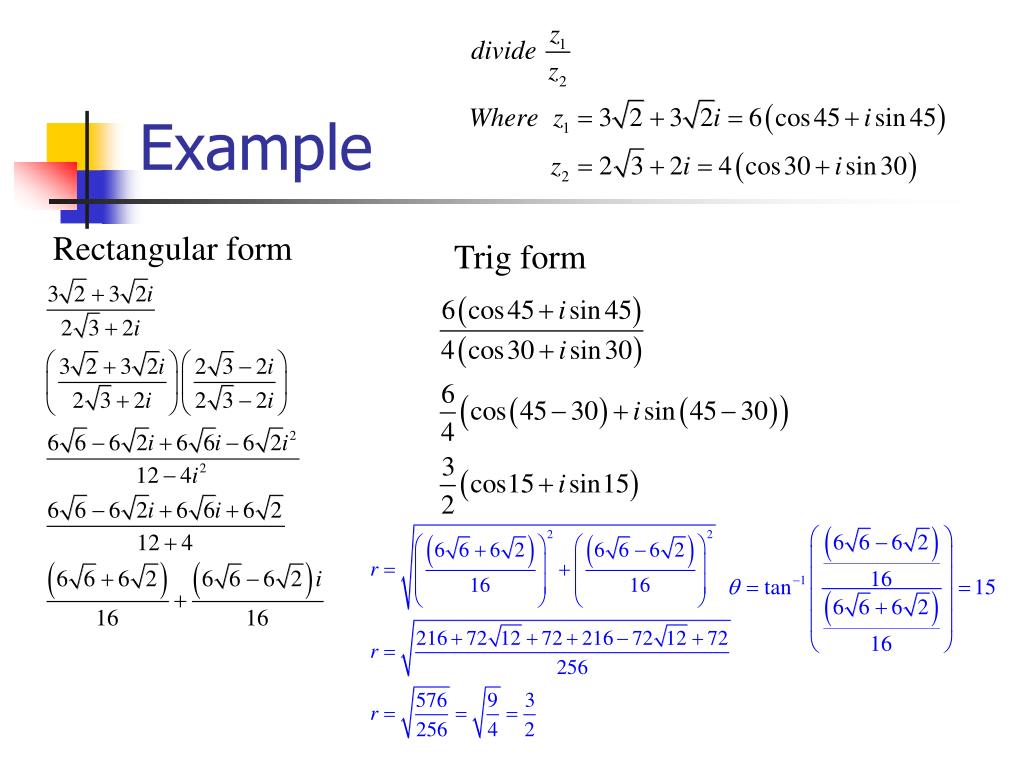
📈Find the quotient of the complex numbers. Express your answer in
Next, we must determine the argument θ. Web the second chapter is devoted to the study of the trigonometric form of complex numbers and it contains two sections dealing with the following aspects: Given a complex number, z = a + b i, we first compute the modulus, r = a 2 + b 2. Web your number, which is.
Express the complex number in trigonometric form. 2 2i
Given a complex number, z = a + b i, we first compute the modulus, r = a 2 + b 2. Distributing to terms inside the (. Z = a+ bi = |z|(cos(θ)+isin(θ)) z = a + b i = | z. Web take the following complex number in rectangular form. Web the answer you will get is:
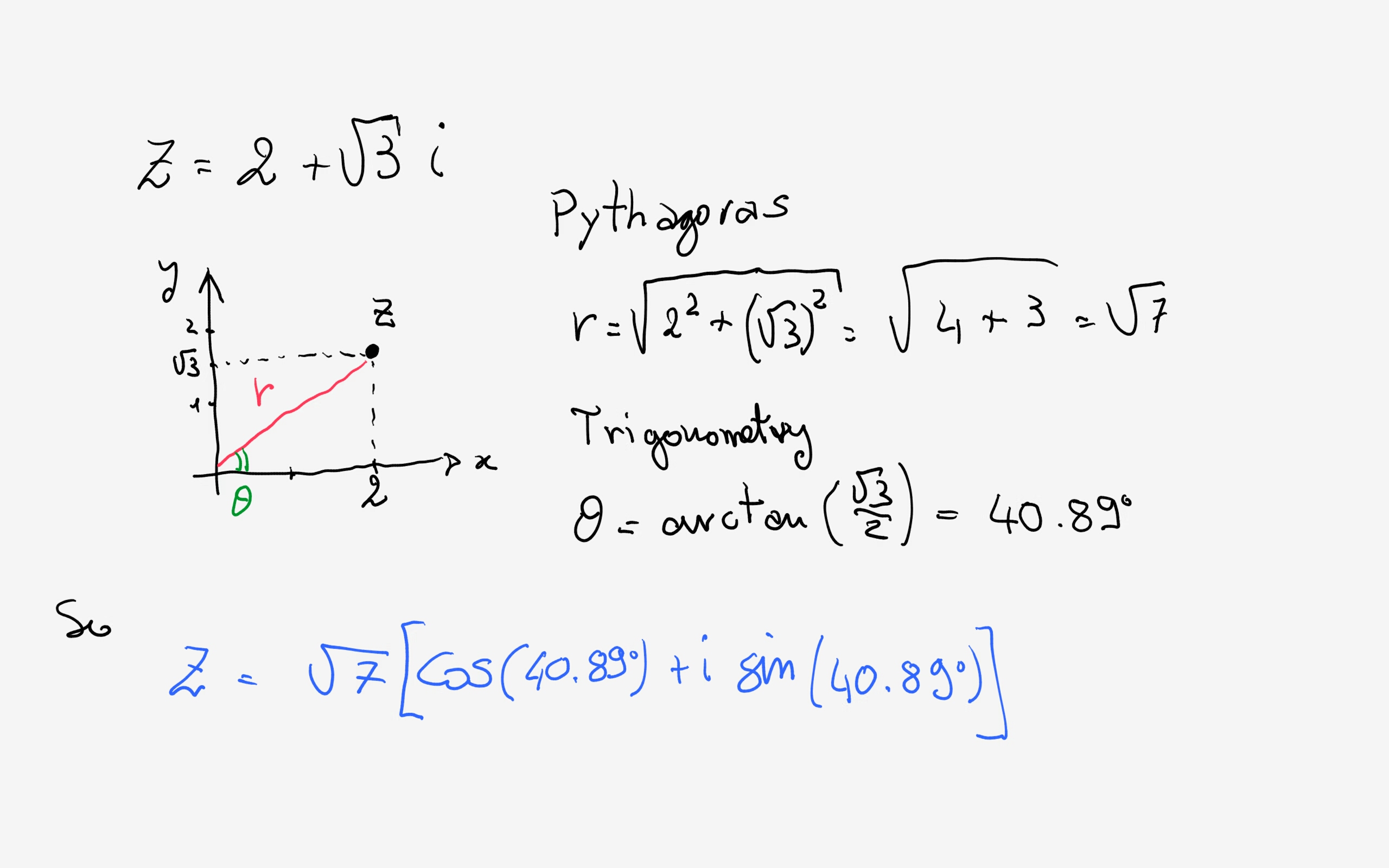
How do you express the complex number in trigonometric form 2+(sqrt 3
Next, we must determine the argument θ. Given a complex number, z = a + b i, we first compute the modulus, r = a 2 + b 2. Web express the complex number in trigonometric form. Web the polar form of a complex number expresses a number in terms of an angle \(\theta\) and its distance from the origin.
PPT Trigonometric Form of a Complex Number PowerPoint Presentation
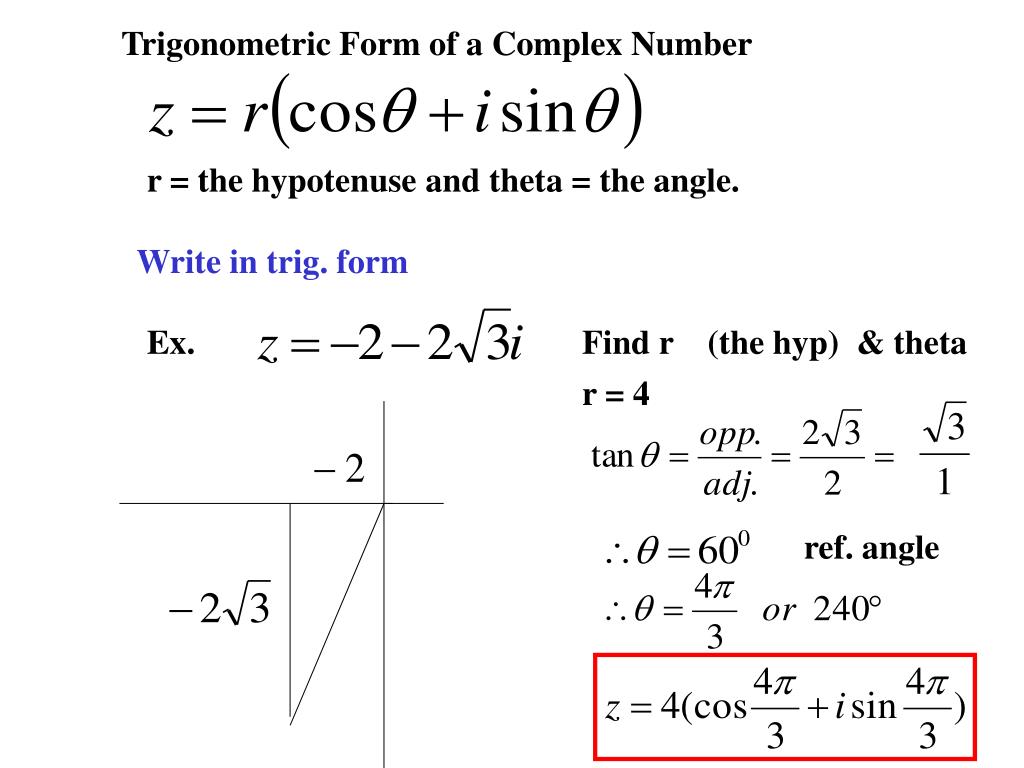
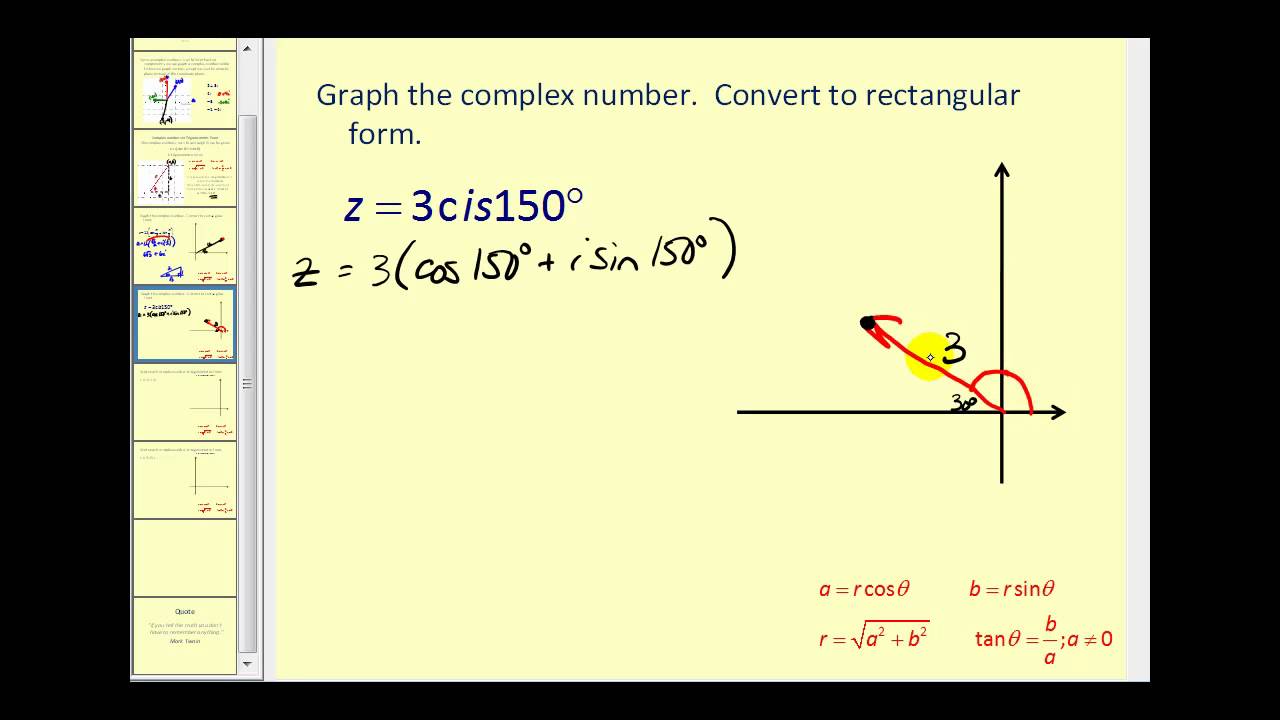
Web the trigonometric form of a complex number z = a + bi is = r(cos i sin ); Here is the graph of 5 + 7 i. Z = a+ bi = |z|(cos(θ)+isin(θ)) z = a + b i = | z. Where r = ja + bij is the modulus of z, and tan we will require 0.
Trigonometric Form Into A Complex Number
Web the second chapter is devoted to the study of the trigonometric form of complex numbers and it contains two sections dealing with the following aspects: Distributing to terms inside the (. Web the cis form of the answer is just another way to write it ( cis(θ) is a symbol that is, by definition, equal to cos(θ) + isin(θ).
Write each complex number in trigonometric form,
Web this is the trigonometric form of a complex number where |z| | z | is the modulus and θ θ is the angle created on the complex plane. Web the answer you will get is: Given a complex number, z = a + b i, we first compute the modulus, r = a 2 + b 2. Web the.
Express the complex number in trigonometric form
Web this is the trigonometric form of a complex number where |z| | z | is the modulus and θ θ is the angle created on the complex plane. Distributing to terms inside the (. \(1−\sqrt{3}i\) to convert the following complex number from rectangular form to trigonometric polar. All of this can also be thought of in terms. Web the.
Complex Numbers in Trigonometric Form YouTube
Web the second chapter is devoted to the study of the trigonometric form of complex numbers and it contains two sections dealing with the following aspects: Given a complex number, z = a + b i, we first compute the modulus, r = a 2 + b 2. Next, we must determine the argument θ. Choose the notation your class.
6.5 Day 1 Trigonometric Form of a Complex Number YouTube
This is the trigonometric form of a complex number where |z| | z | is the modulus and θ θ is the angle created on the. Web express the complex number in trigonometric form. 6 (cos (2pi/3) + i sin (2pi/3)) arrow right. To convert a complex number z to trigonometric form, we use the formula: Web the second chapter.
Web Take The Following Complex Number In Rectangular Form.
Given a complex number, z = a + b i, we first compute the modulus, r = a 2 + b 2. Web the answer you will get is: Web how to write complex numbers in trigonometric form? = b is called the argument of z.
As A Refresher, The Distance Between The Origin And The Complex Number Is Equal To $|A + Bi| = \Sqrt{A^2 + B^2}$.
All of this can also be thought of in terms. Next, we must determine the argument θ. Web how do you express the complex number in trigonometric form: Distributing to terms inside the (.
Here Is The Graph Of 5 + 7 I.
A few ways to express this is: Precalculus complex numbers in trigonometric form trigonometric form of. Web express the complex number in trigonometric form. Z = a+ bi = |z|(cos(θ)+isin(θ)) z = a + b i = | z.
Web This Is The Trigonometric Form Of A Complex Number Where |Z| | Z | Is The Modulus And Θ Θ Is The Angle Created On The Complex Plane.
Where r = ja + bij is the modulus of z, and tan we will require 0 < 2. Web the cis form of the answer is just another way to write it ( cis(θ) is a symbol that is, by definition, equal to cos(θ) + isin(θ) ). Converting to polar from rectangular, x = 5 and y = 7. R = 5 2 + 7 2 =.