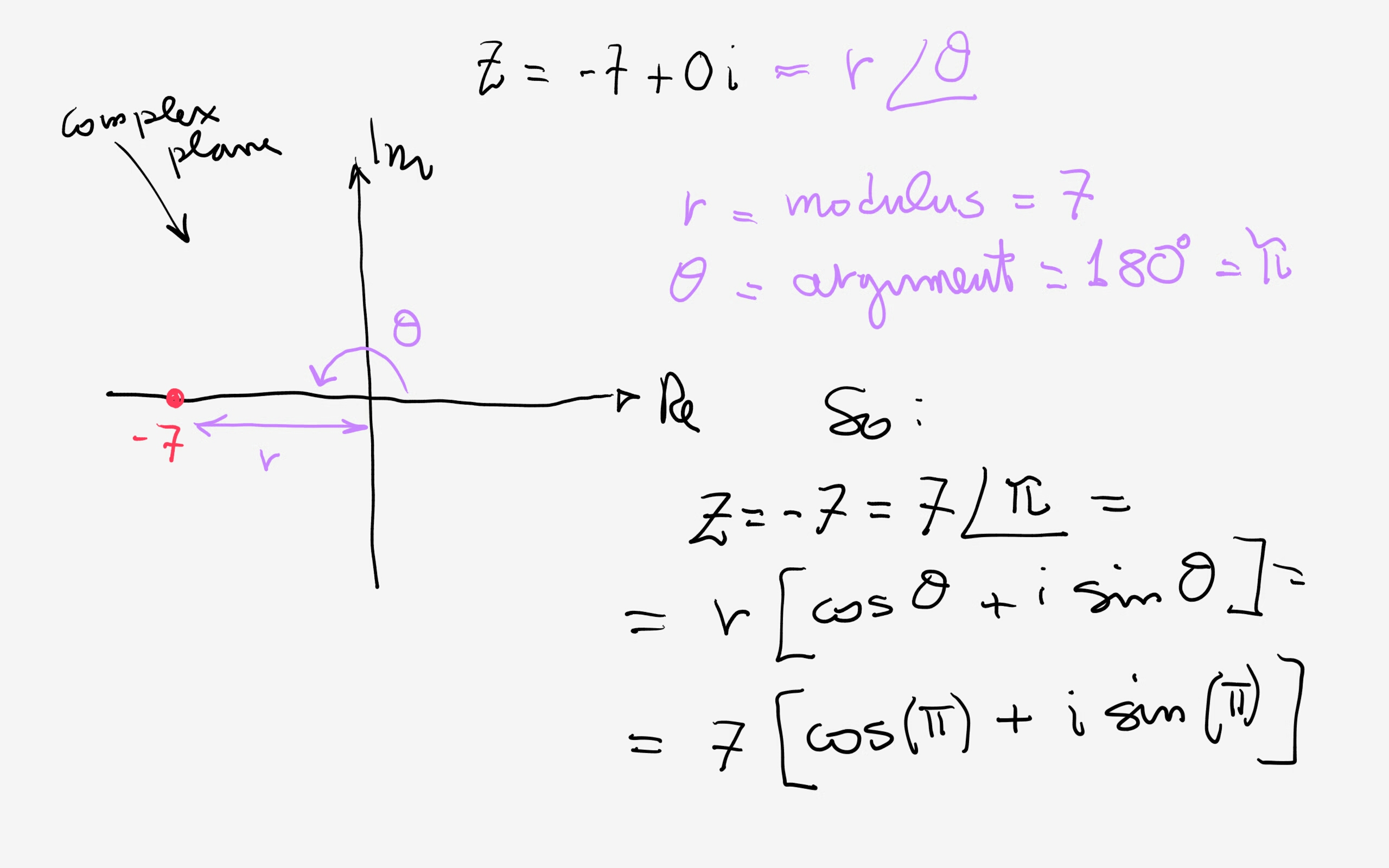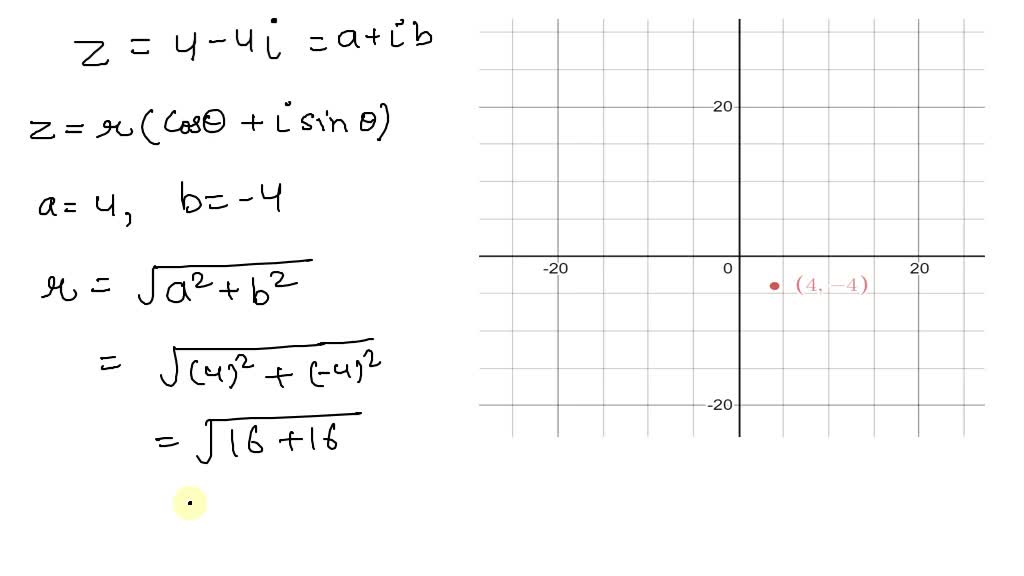Complex Number To Trigonometric Form
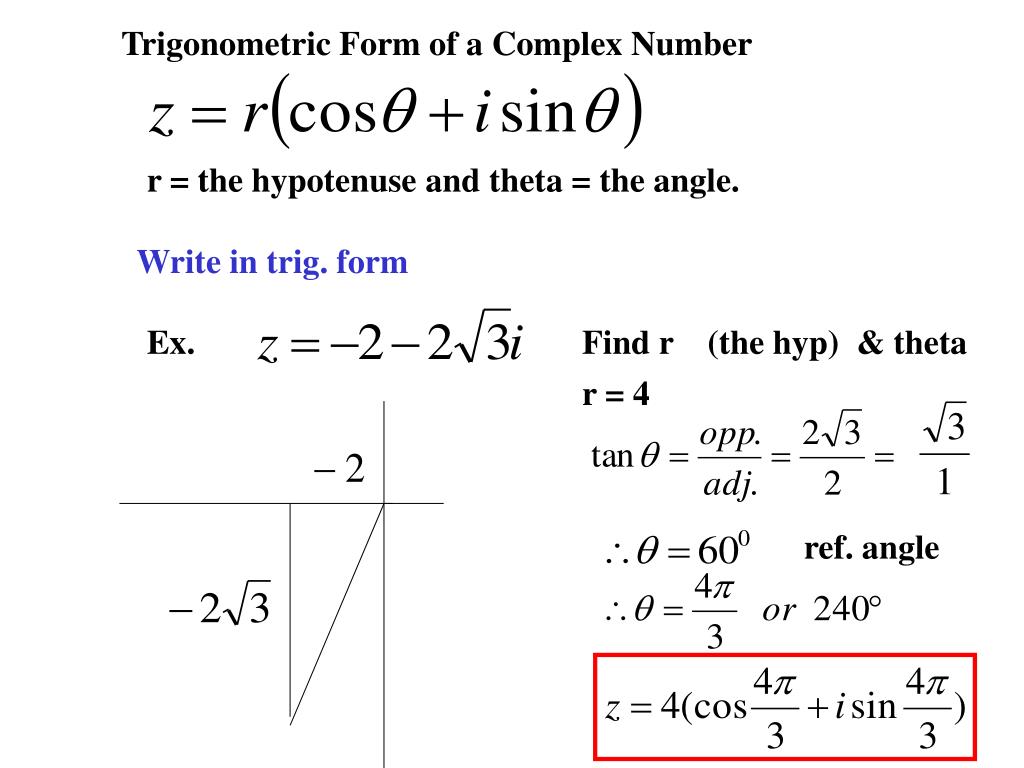
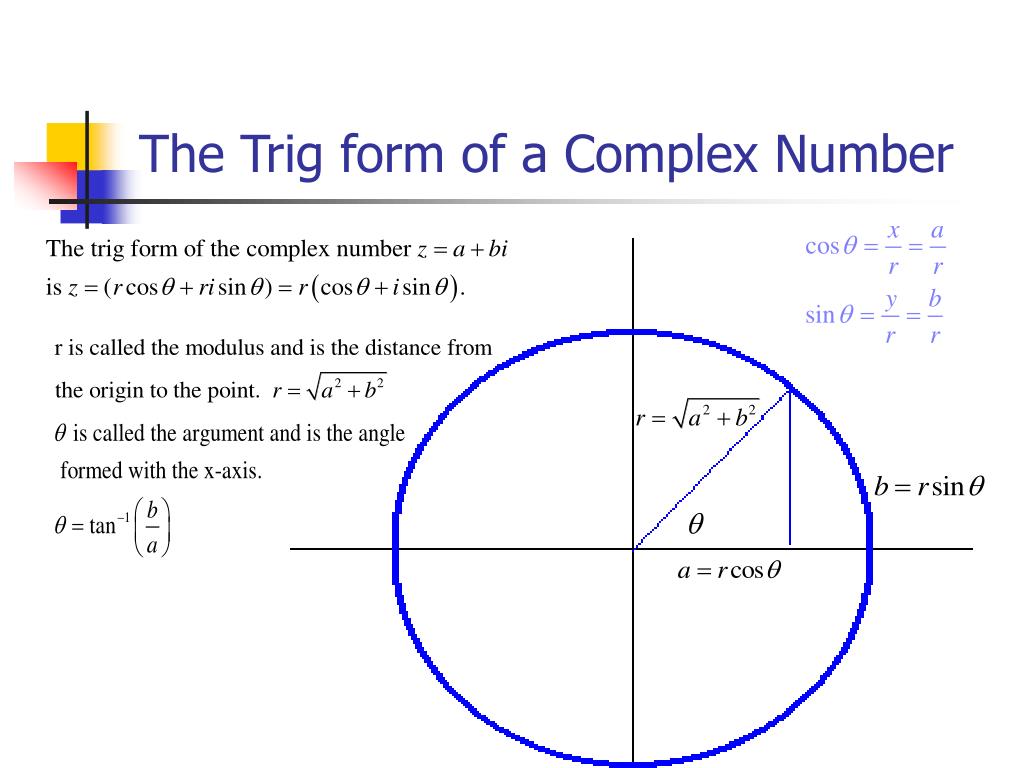
Complex Number To Trigonometric Form - Expression where is called trigonometric form of complex number. From the rectangular form of − 3.12 − 4.64 i x = − 3.12 and y = − 4.64. $z = r(\cos \alpha + i\cdot. = a + bi becomes z = r(cos + isin ) = |z| and the reference angle, ' is given by tan ' = |b/a| note that it is up to you to make sure is in the. = b is called the argument of z. 88(cos π + i sin π) \(5\left(\cos. Where r = ja + bij is the modulus of z, and tan we will require 0 < 2. Given a +ib, let r =. \(1−\sqrt{3}i\) to convert the following complex number from rectangular form to trigonometric polar form,. Web find the absolute value of the complex numbers.
Web convert a complex number into trigonometric form. From the rectangular form of − 3.12 − 4.64 i x = − 3.12 and y = − 4.64. = b is called the argument of z. Web depending on what you need to do with your complex numbers, the trigonometric form can be very useful or very thorny. Web this is the trigonometric form of a complex number where |z| | z | is the modulus and θ θ is the angle created on the complex plane. Web euler's formula states that for any real number x : A complex number is a number that. Web trigonometric form of complex numbers. Where r = ja + bij is the modulus of z, and tan we will require 0 < 2. Except for $0,$ any complex number can be represented in the trigonometric form or in polar coordinates:
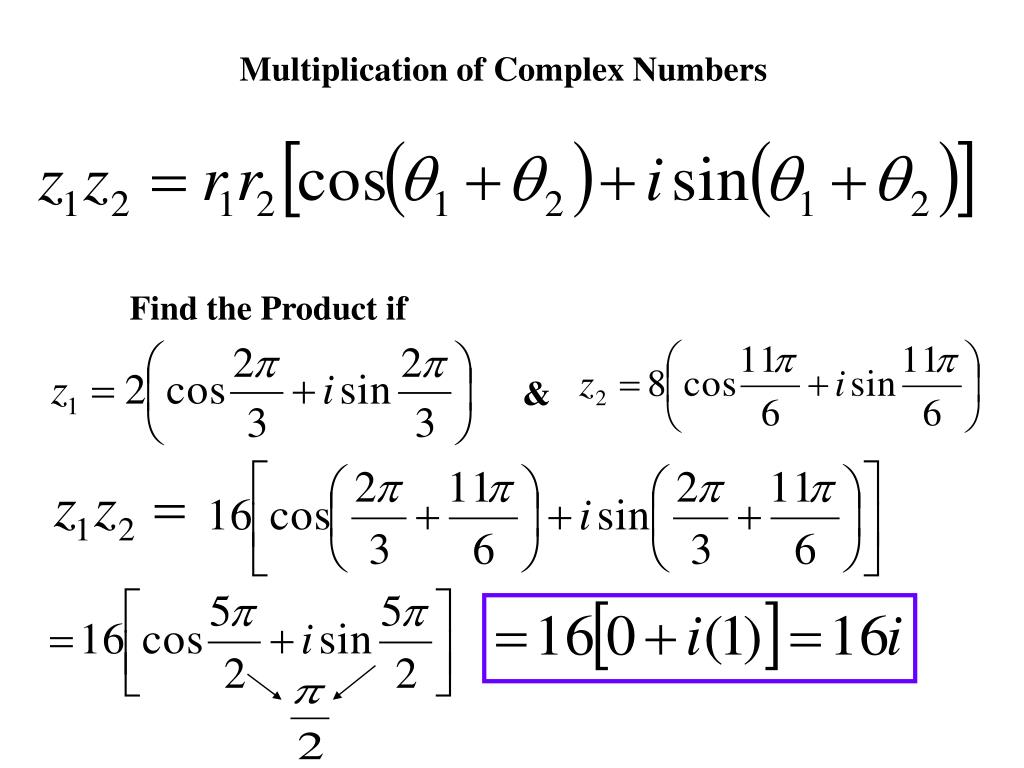
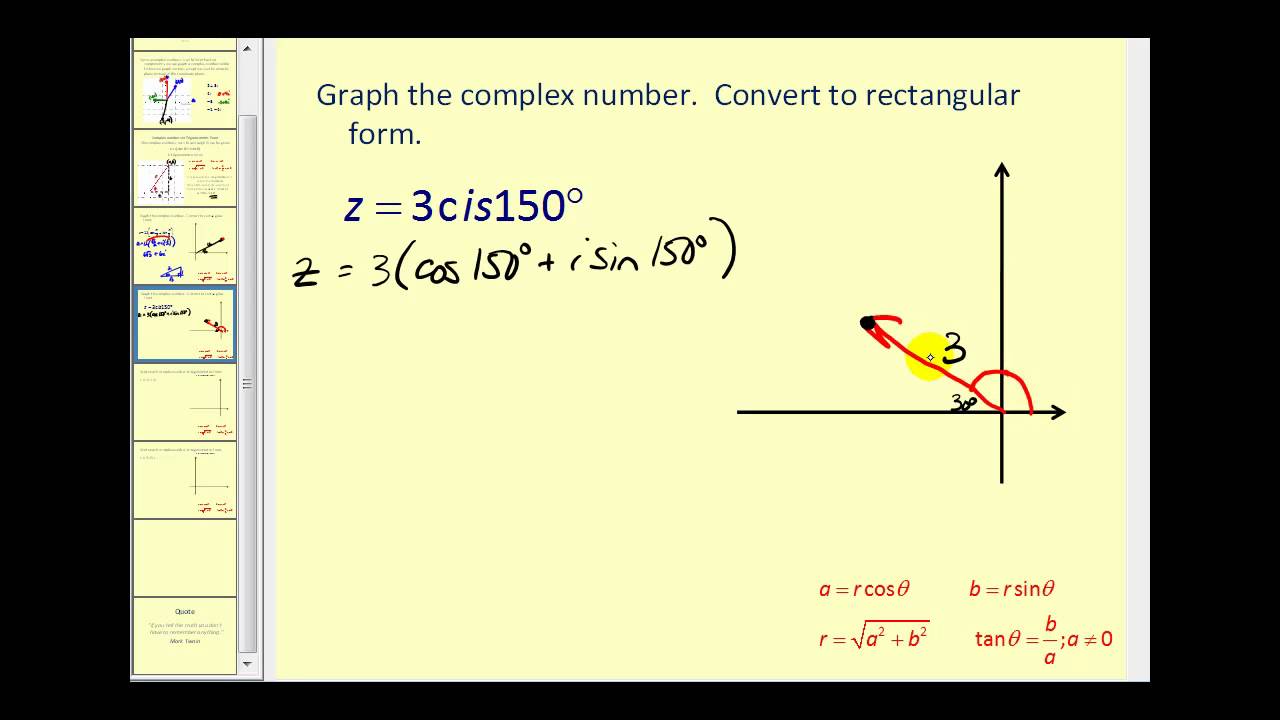
\(1−\sqrt{3}i\) to convert the following complex number from rectangular form to trigonometric polar form,. This is the trigonometric form of a. Web the trigonometric form of a complex number provides a relatively quick and easy way to compute products of complex numbers. Web the general trigonometric form of complex numbers is r ( cos θ + i sin θ). Web find the absolute value of the complex numbers. From the rectangular form of − 3.12 − 4.64 i x = − 3.12 and y = − 4.64. For example, let z1 = 1 + i, z2 = √3 +i and z3 = −1 +i√3. Z = a + b i = r ( cos θ + i sin θ), where we usually require that 0 ≤ θ ≤ 2 π. A complex number is a number that. Except for $0,$ any complex number can be represented in the trigonometric form or in polar coordinates:
Trigonometric Form Into A Complex Number
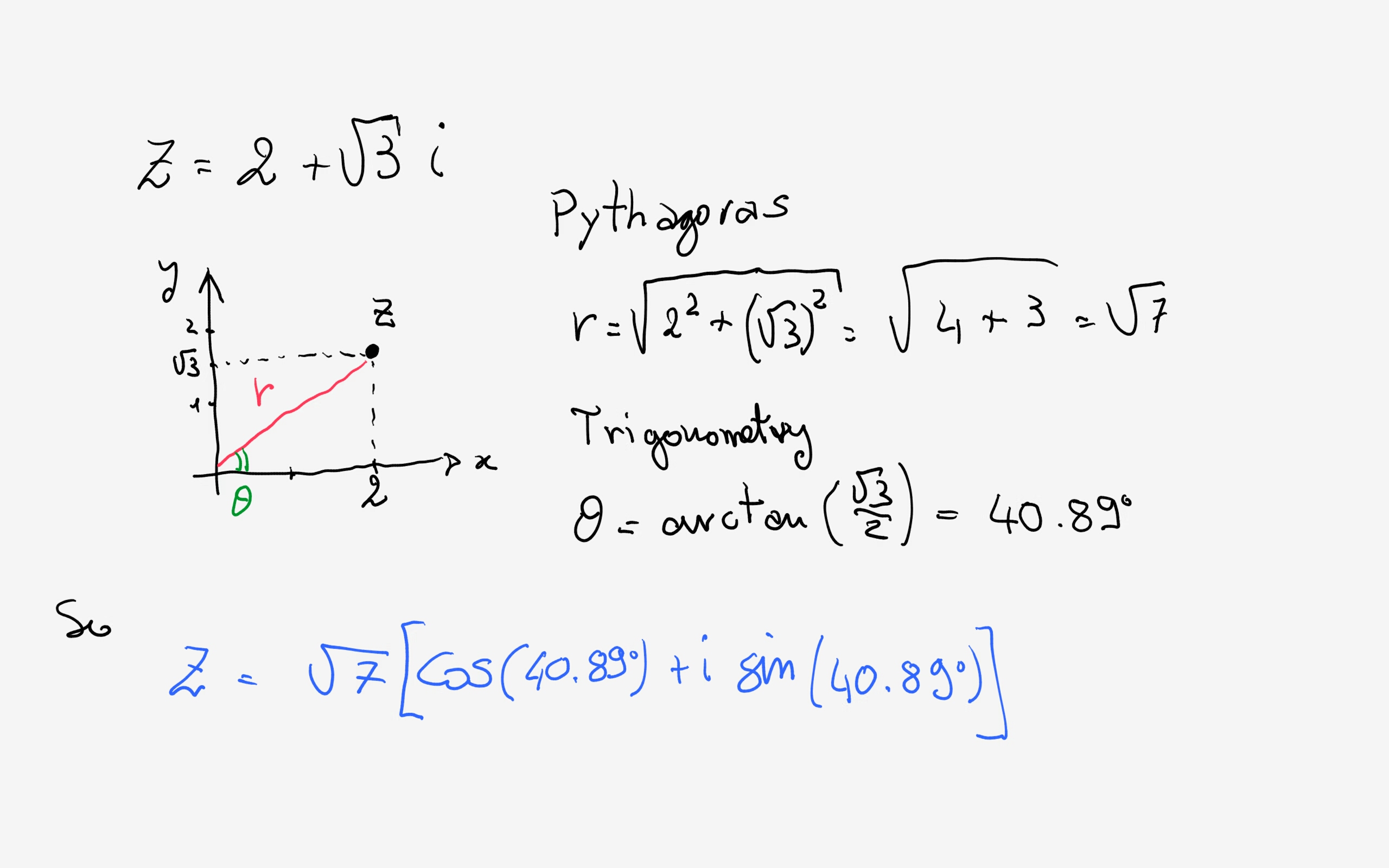
For example, let z1 = 1 + i, z2 = √3 +i and z3 = −1 +i√3. Z = a+ bi = |z|(cos(θ)+isin(θ)) z = a + b i = | z. Where e is the base of the natural logarithm, i is the imaginary unit, and cos and sin are the trigonometric functions cosine. Z = a + b.
PPT 6.5 Trig. Form of a Complex Number PowerPoint Presentation, free
9.1 verifying trigonometric identities and using trigonometric identities to simplify trigonometric expressions;. = a + bi becomes z = r(cos + isin ) = |z| and the reference angle, ' is given by tan ' = |b/a| note that it is up to you to make sure is in the. Web the trigonometric form of a complex number provides a.
How do you express the complex number in trigonometric form 2+(sqrt 3
As a consequence, we will be able. This is the trigonometric form of a. Web represent the complex number − 3.12 − 4.64 i graphically and give two notations of its polar form. Expression where is called trigonometric form of complex number. = b is called the argument of z.
Multiplying Complex Numbers In Trigonometric Form YouTube
Web introduction to trigonometric identities and equations; Web convert a complex number into trigonometric form. Web represent the complex number − 3.12 − 4.64 i graphically and give two notations of its polar form. As a consequence, we will be able. Asked 8 years, 9 months ago.
The Product and Quotient of Complex Numbers in Trigonometric Form YouTube
Web the trigonometric form of a complex number provides a relatively quick and easy way to compute products of complex numbers. Web take the following complex number in rectangular form. = a + bi becomes z = r(cos + isin ) = |z| and the reference angle, ' is given by tan ' = |b/a| note that it is up.
PPT Trigonometric Form of a Complex Number PowerPoint Presentation
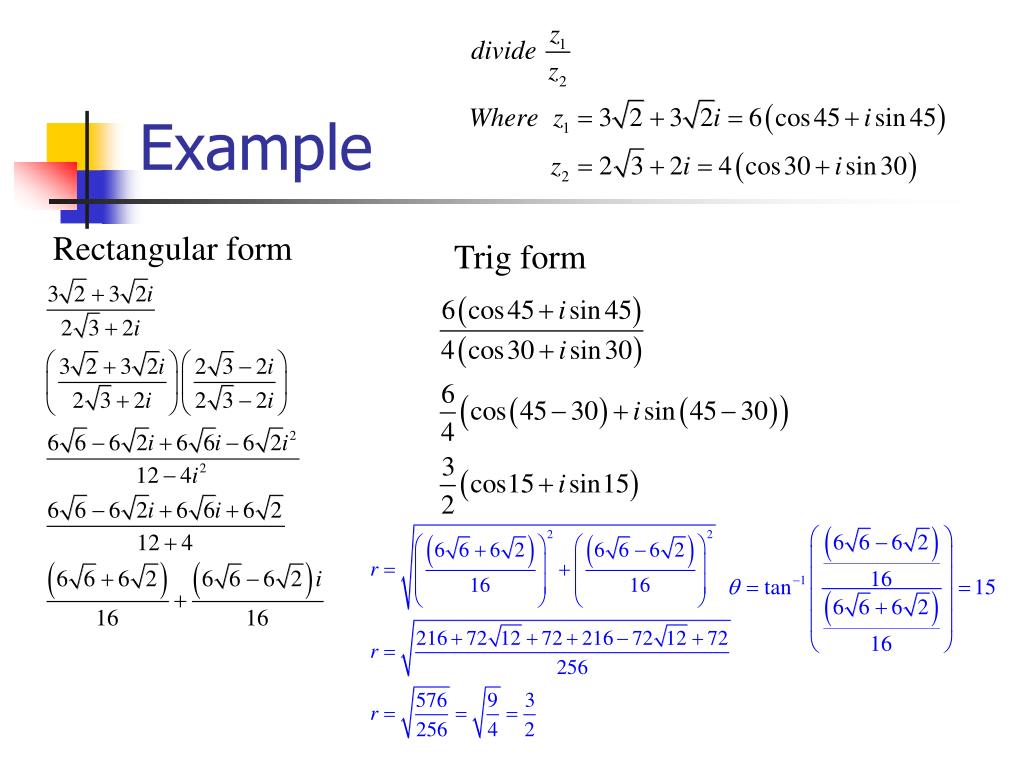
Where e is the base of the natural logarithm, i is the imaginary unit, and cos and sin are the trigonometric functions cosine. = b is called the argument of z. Web to multiply two complex numbers z1 = a + bi and z2 = c + di, use the formula: $z = r(\cos \alpha + i\cdot. Web convert a.
PPT Trigonometric Form of a Complex Number PowerPoint Presentation
Web trigonometric form of complex numbers. = a + bi becomes z = r(cos + isin ) = |z| and the reference angle, ' is given by tan ' = |b/a| note that it is up to you to make sure is in the. By nature of complex numbers, this. If you express your complex number in polar form as.
How do you write the complex number in trigonometric form 7? Socratic
Web the trigonometric form of a complex number provides a relatively quick and easy way to compute products of complex numbers. Web euler's formula states that for any real number x : 9.1 verifying trigonometric identities and using trigonometric identities to simplify trigonometric expressions;. Web represent the complex number − 3.12 − 4.64 i graphically and give two notations of.
Complex Numbers in Trigonometric Form YouTube
Where r = ja + bij is the modulus of z, and tan we will require 0 < 2. Modified 8 years, 9 months ago. For example, let z1 = 1 + i, z2 = √3 +i and z3 = −1 +i√3. If you express your complex number in polar form as r(cosθ +isinθ), then it has fourth roots: Web.
SOLVEDTrigonometric Form of a Complex Number Rep…
= b is called the argument of z. This is the trigonometric form of a. The trigonometric form of “x + yi” is r(cos θ + i sin θ). From the rectangular form of − 3.12 − 4.64 i x = − 3.12 and y = − 4.64. Web to multiply two complex numbers z1 = a + bi and.
9.1 Verifying Trigonometric Identities And Using Trigonometric Identities To Simplify Trigonometric Expressions;.
Except for $0,$ any complex number can be represented in the trigonometric form or in polar coordinates: Web trigonometric form of a complex number. Asked 8 years, 9 months ago. Web the trigonometric form of a complex number provides a relatively quick and easy way to compute products of complex numbers.
Where R = Ja + Bij Is The Modulus Of Z, And Tan We Will Require 0 < 2.
= b is called the argument of z. \(1−\sqrt{3}i\) to convert the following complex number from rectangular form to trigonometric polar form,. What is a complex number? $z = r(\cos \alpha + i\cdot.
Web This Is The Trigonometric Form Of A Complex Number Where |Z| | Z | Is The Modulus And Θ Θ Is The Angle Created On The Complex Plane.
Α = 4√r(cos(θ 4) +isin( θ 4)), iα, −α and −iα explanation: Web depending on what you need to do with your complex numbers, the trigonometric form can be very useful or very thorny. This is the trigonometric form of a. Web take the following complex number in rectangular form.
Web Introduction To Trigonometric Identities And Equations;
Web the general trigonometric form of complex numbers is r ( cos θ + i sin θ). Modified 8 years, 9 months ago. A complex number is a number that. For example, let z1 = 1 + i, z2 = √3 +i and z3 = −1 +i√3.