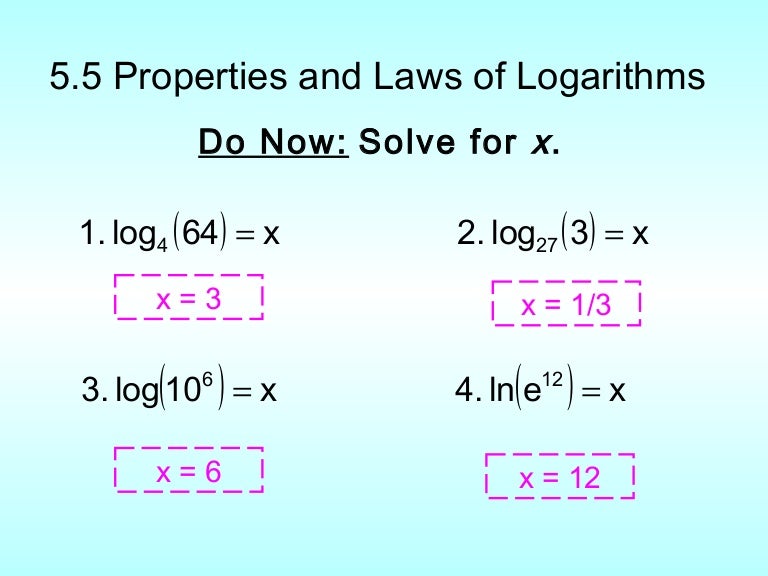
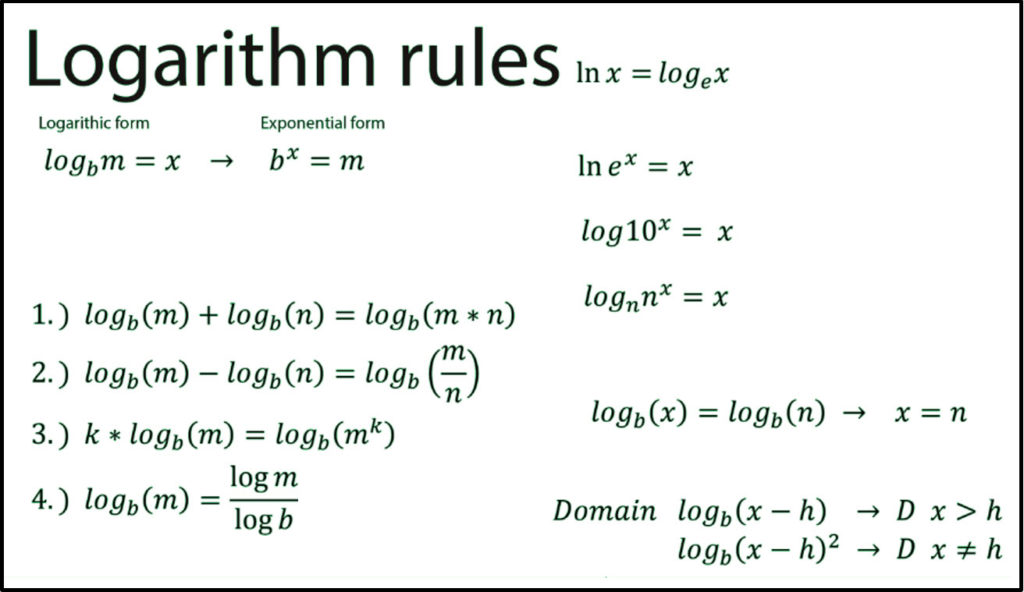
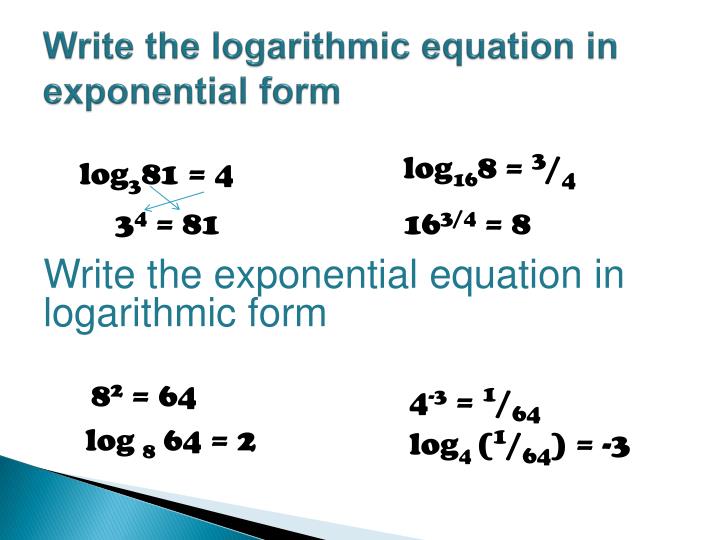
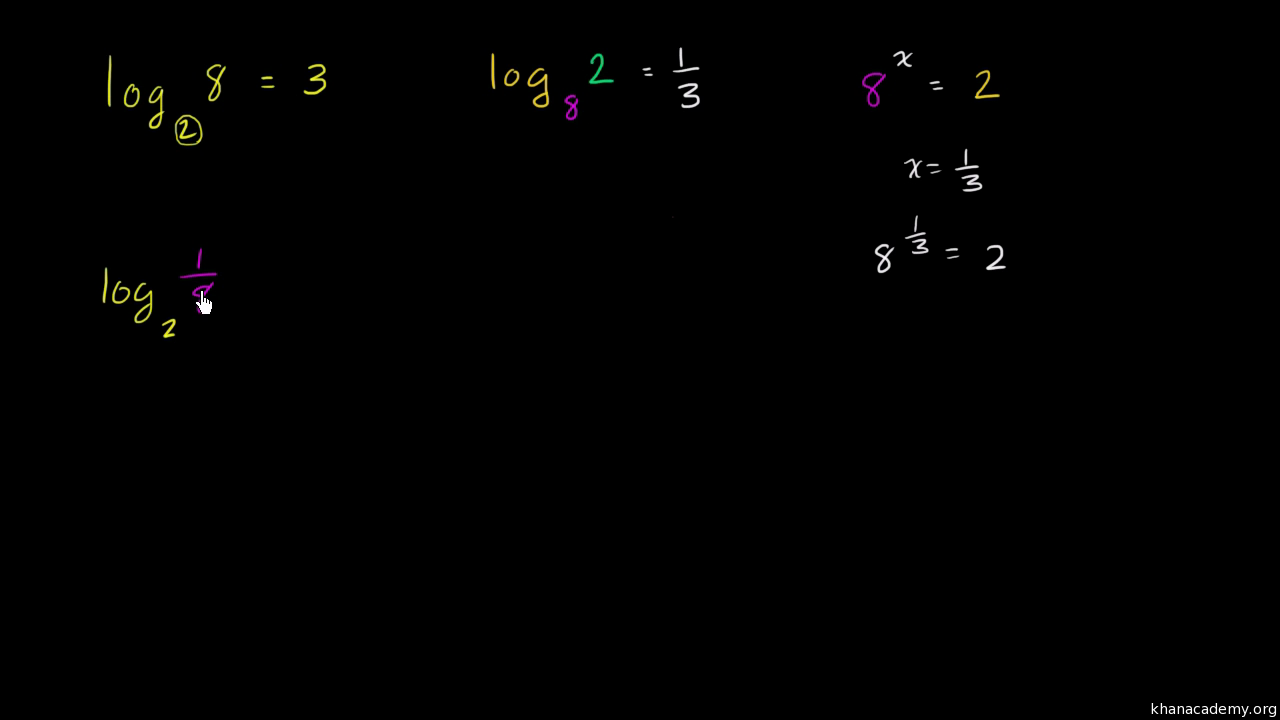3 4 81 In Logarithmic Form
3 4 81 In Logarithmic Form - Please help me write in logarithmic form 3^4 = 81 this question is from textbook answer by nate(3500) ( show source ): Web logarithm form 3^4= 81. You'll get a detailed solution from a subject matter expert that helps you learn. For logarithmic equations, logb(x) = y log b ( x) = y is equivalent to by = x b y = x such that x > 0 x > 0, b. In our case, a = 3, b = 81, and x = 4. Write the given expression in logarithmic form. In this segment we will cover equations with logarithms. Web the equation 3^(4)=81 rewritten in logarithmic form, would be this problem has been solved! Convert to logarithmic form 81=3^4. The logarithm calculator simplifies the given logarithmic expression by using the laws of logarithms.
Please help me write in logarithmic form 3^4 = 81 this question is from textbook answer by nate(3500) ( show source ): For logarithmic equations, logb(x) = y log b ( x) = y is equivalent to by = x b y = x such that x > 0 x > 0, b. Therefore, our equation in exponential form would. In this segment we will cover equations with logarithms. One logarithmic rule states that logab = x is the same as ax = b. You'll get a detailed solution from a subject matter expert that helps you learn. Convert to logarithmic form 81=3^4. Convert the exponential equation to a logarithmic equation using the logarithm base (3) ( 3) of the left side (81) (. Log3 (81) = 4 log 3 ( 81) = 4. X^ {\msquare} \log_ {\msquare} \sqrt {\square} \nthroot [\msquare] {\square} \le.
The logarithm calculator simplifies the given logarithmic expression by using the laws of logarithms. You'll get a detailed solution from a subject matter expert that helps you learn. In our case, a = 3, b = 81, and x = 4. Convert to logarithmic form 81=3^4. X^ {\msquare} \log_ {\msquare} \sqrt {\square} \nthroot [\msquare] {\square} \le. Please help me write in logarithmic form 3^4 = 81 this question is from textbook answer by nate(3500) ( show source ): Convert the exponential equation to a logarithmic equation using the logarithm base (3) ( 3) of the left side (81) (. You can put this solution on your website! Web write 3 4 = 81 in logarithmic form. 81 = 34 81 = 3 4.
Write an Exponential 3^4=81 in Logarithmic Form (Definition of Log
Therefore, our equation in exponential form would. Convert the exponential equation to a logarithmic equation using the logarithm base (3) ( 3) of the left side (81) (. Please help me write in logarithmic form 3^4 = 81 this question is from textbook answer by nate(3500) ( show source ): One logarithmic rule states that logab = x is the.
Logarithms
Enter the logarithmic expression below which you want to simplify. Therefore, our equation in exponential form would. Web 3^4=81 pre algebra algebra pre calculus calculus functions linear algebra trigonometry statistics physics chemistry finance economics conversions go examples related. You'll get a detailed solution from a subject matter expert that helps you learn. Web write in exponential form log base 3.
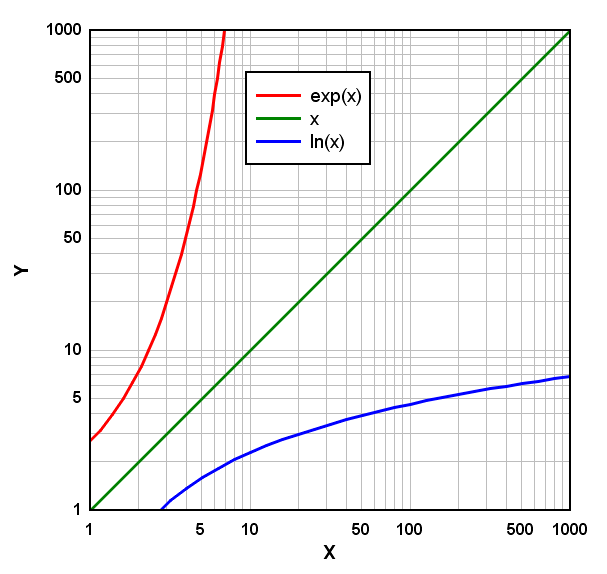
DPlot Logarithmic Scale
Web the equation 3^(4)=81 rewritten in logarithmic form, would be this problem has been solved! You'll get a detailed solution from a subject matter expert that helps you learn. In this segment we will cover equations with logarithms. Please help me write in logarithmic form 3^4 = 81 this question is from textbook answer by nate(3500) ( show source ):.
9. Write the following in logarithmic form 3^4 = 81 YouTube
Web logarithm form 3^4= 81. 81 = 34 81 = 3 4. Convert the exponential equation to a logarithmic equation using the logarithm base (3) ( 3) of the left side (81) (. \displaystyle{3}\cdot{3}\cdot{3}\cdot{3}={81} how do you write the equation \displaystyle{{\log}_{{3}}{81}}={4} in exponential form?. X^ {\msquare} \log_ {\msquare} \sqrt {\square} \nthroot [\msquare] {\square} \le.
7 3 Study Guide And Intervention Logarithms And Logarithmic Functions
Web the equation 3^(4)=81 rewritten in logarithmic form, would be this problem has been solved! Convert the exponential equation to a logarithmic equation using the logarithm base (3) ( 3) of the. 81 = 34 81 = 3 4. Web logarithm form 3^4= 81. You'll get a detailed solution from a subject matter expert that helps you learn.
👍 Which logarithmic equation is equivalent to 32 9. SOLUTION write 32
Therefore, our equation in exponential form would. Web 3^4=81 pre algebra algebra pre calculus calculus functions linear algebra trigonometry statistics physics chemistry finance economics conversions go examples related. Log3 (81) = 4 log 3 ( 81) = 4. One logarithmic rule states that logab = x is the same as ax = b. For logarithmic equations, logb(x) = y log.
How To Write Logarithms In Exponential Form The definition of a
Write the expression in logarithmic form. For logarithmic equations, logb(x) = y log b ( x) = y is equivalent to by = x b y = x such that x > 0 x > 0, b. Web 3^4=81 pre algebra algebra pre calculus calculus functions linear algebra trigonometry statistics physics chemistry finance economics conversions go examples related. Write the.
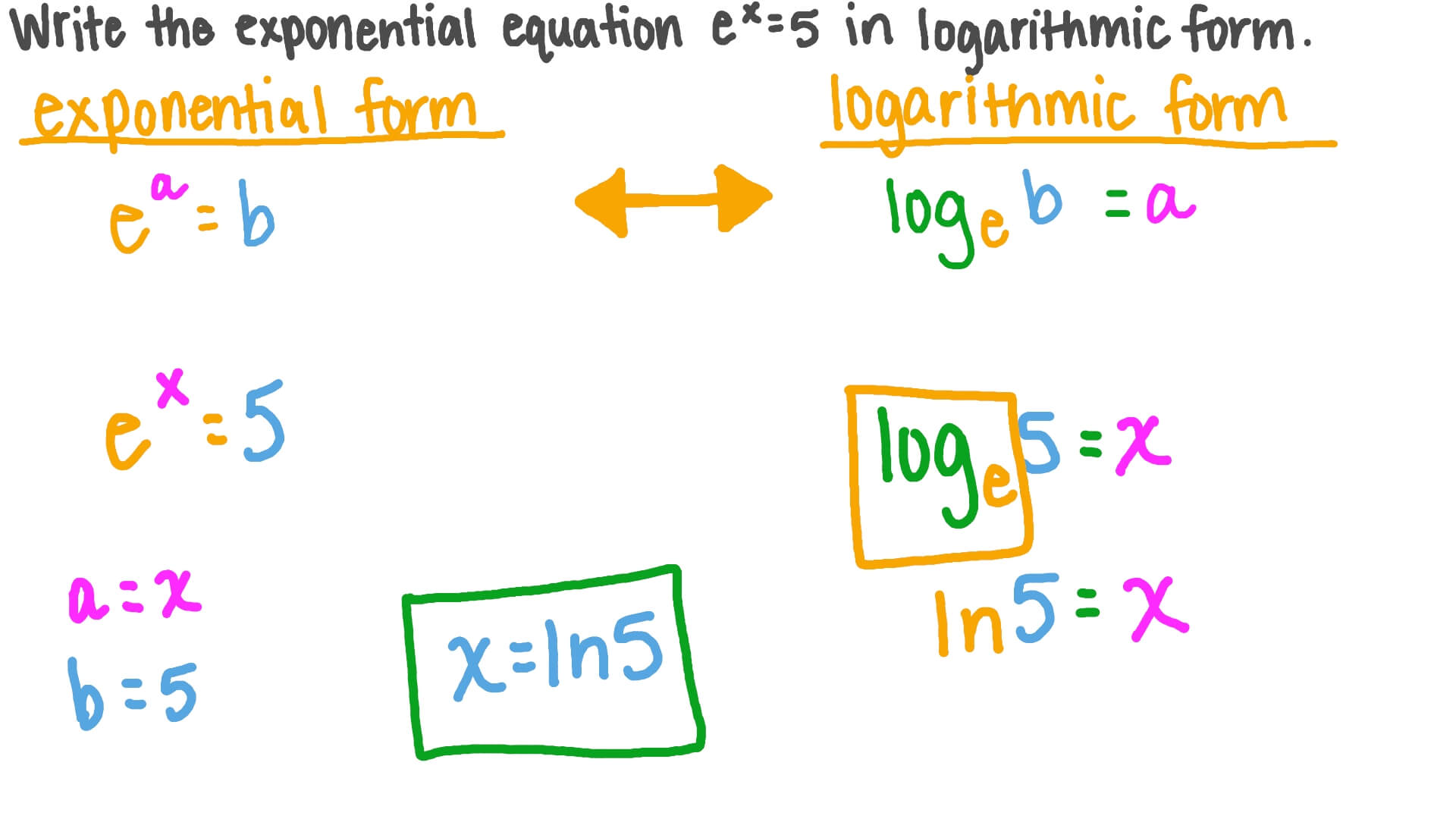
Question Video Rewriting an Exponential Equation in Logarithmic Form
Web the equation 3^(4)=81 rewritten in logarithmic form, would be this problem has been solved! In this segment we will cover equations with logarithms. Write the given expression in logarithmic form. Therefore, our equation in exponential form would. For logarithmic equations, logb(x) = y log b ( x) = y is equivalent to by = x b y = x.
Logarithms
Web write 3 4 = 81 in logarithmic form. In our case, a = 3, b = 81, and x = 4. Log3 (81) = 4 log 3 ( 81) = 4. Please help me write in logarithmic form 3^4 = 81 this question is from textbook answer by nate(3500) ( show source ): Write the given expression in logarithmic.
PPT 5.2 Logarithmic Functions & Their Graphs PowerPoint Presentation
In this segment we will cover equations with logarithms. The logarithm calculator simplifies the given logarithmic expression by using the laws of logarithms. Convert the exponential equation to a logarithmic equation using the logarithm base (3) ( 3) of the left side (81) (. Therefore, our equation in exponential form would. Web the equation 3^(4)=81 rewritten in logarithmic form, would.
You'll Get A Detailed Solution From A Subject Matter Expert That Helps You Learn.
Write the expression in logarithmic form. For logarithmic equations, logb(x) = y log b ( x) = y is equivalent to by = x b y = x such that x > 0 x > 0, b. Web write 3 4 = 81 in logarithmic form. Web logarithm form 3^4= 81.
Web 3^4=81 Pre Algebra Algebra Pre Calculus Calculus Functions Linear Algebra Trigonometry Statistics Physics Chemistry Finance Economics Conversions Go Examples Related.
81 = 34 81 = 3 4. Log3 (81) = 4 log 3 ( 81) = 4. The logarithm calculator simplifies the given logarithmic expression by using the laws of logarithms. In our case, a = 3, b = 81, and x = 4.
Therefore, Our Equation In Exponential Form Would.
You can put this solution on your website! Convert to logarithmic form 81=3^4. Web write in exponential form log base 3 of 81=4. X^ {\msquare} \log_ {\msquare} \sqrt {\square} \nthroot [\msquare] {\square} \le.
Please Help Me Write In Logarithmic Form 3^4 = 81 This Question Is From Textbook Answer By Nate(3500) ( Show Source ):
Write the given expression in logarithmic form. \displaystyle{3}\cdot{3}\cdot{3}\cdot{3}={81} how do you write the equation \displaystyle{{\log}_{{3}}{81}}={4} in exponential form?. Enter the logarithmic expression below which you want to simplify. Convert the exponential equation to a logarithmic equation using the logarithm base (3) ( 3) of the left side (81) (.